ベクトル積分と置換積分公式 


3次元空間でのベクトル積分と言えば、線積分 \Int[R] \b F \sx d\b r
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \b F \sx d\b r } $
l.33 $}
、面積分 \Int[S] \b F \sx d\b S
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \b F \sx d\b S } $
l.33 $}
、体積分 \Int[V] F \, d V
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] F \, d V } $
l.33 $}
。これらを結びつける置換積分公式として、ストークスの定理 \Int[R] \b F \sx d\b r
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \b F \sx d\b r } $
l.33 $}
= \Int[S] \nabla \vx \b F \sx d\b S
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \nabla \vx \b F \sx d\b S } $
l.33 $}
とガウスの定理 \Int[S] \b F \sx d\b S
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \b F \sx d\b S } $
l.33 $}
= \Int[V] \nabla \sx \b F \, dV
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \nabla \sx \b F \, dV } $
l.33 $}
がある。
名前の「線」「面」「体」は伊達ではなく、これらの公式はある種の「次元」を超えている。大抵の説明は複雑な図と面倒な成分計算を使うが、それは通常のベクトル計算では「次元」超えに無理があるためで、微分形式と外積代数を使えば、もっと効率良く扱える。しかし、記号の衝突があって、ベクトルと外積代数の橋渡しに苦労が多い。
ベクトル積分をショートカットするには外積代数を使うべき。そのためにはベクトル演算と外積代数の演算を両立させる表記が必要。話しが多分野に渡り、大きくなるため、置換積分の話しは数回に分割する。初回は通常のベクトル演算のみで頑張り、外積代数は次回から導入する。
今回作戦は、まず、ベクトル微分演算子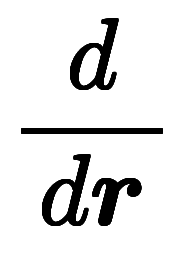 と同様、ベクトル積分を機能別に分解して表記。これよりガウスの定理は偶然にも計算できてしまうが、ストークスの定理では破綻する。このため、イカサマ規則を1つ加えて、無理やりに計算できるようにさせる。
と同様、ベクトル積分を機能別に分解して表記。これよりガウスの定理は偶然にも計算できてしまうが、ストークスの定理では破綻する。このため、イカサマ規則を1つ加えて、無理やりに計算できるようにさせる。
ベクトル積分演算子 


ベクトル微分演算子の時と同様、表記を機能毎に独立させた方が操作しやすい。ベクトル積分の場合は、ベクトル、積分、範囲の3つの機能を併せ持つ。 \Int[R] \b F \sx d\b r
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \b F \sx d\b r } $
l.33 $}
では、 でベクトル、
でベクトル、 \Int[R] \
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ } $
l.33 $}
で積分と範囲の両方を表している。厳密には が積分、
が積分、 が範囲を表しているが、記号としては分離できない。
が範囲を表しているが、記号としては分離できない。
積分の演算子表記 


猫式では \Int[R] \
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ } $
l.33 $}
を範囲指定の専用記号とした。範囲の指定は や
や などとバリエーションが多く、従来表記と楽に相互変換するために元のまま残したい。それに、積分を表す記号は別に当てがある。
などとバリエーションが多く、従来表記と楽に相互変換するために元のまま残したい。それに、積分を表す記号は別に当てがある。
1次元の場合、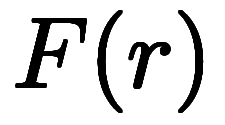 =
=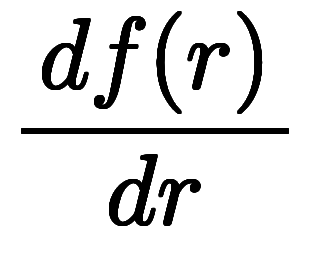 ⇔
⇔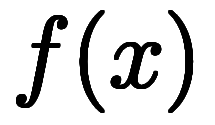 =
= \Int F(x)\,dx
/home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
F(x)\,dx } $
l.33 $}
。分数計算の感覚で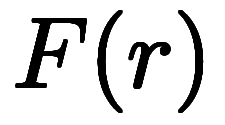 =
=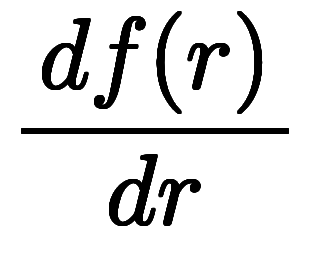 を
を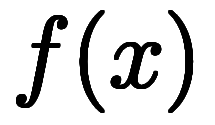 について解くと、
について解くと、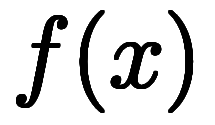 =
=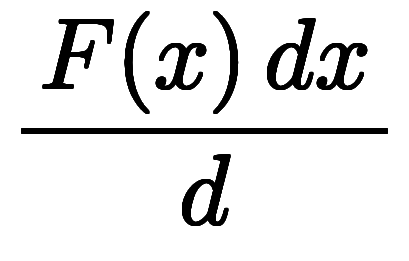 と表現できる*1。したがって、猫式では
と表現できる*1。したがって、猫式では で積分を表し、不定積分は
で積分を表し、不定積分は が付かない。
が付かない。
また、微分演算子のように、式変形が便利のように以下の書式も許す。
| 分数形 | 演算子形 |
|---|
\Int[R] \ffd{\b F\,dx}{d} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ffd {\b F\,dx}{d} } $
l.33 $}
| \Int[R] \ffd{dx}{d} \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ffd {dx}{d} \b F } $
l.33 $}
| \Int[R] d^-\!\!dx\,\b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] d^-\!\!dx\,\b F } $
l.33 $}
|
積分の階数表記 


3次元における線積分、面積分、体積分の微小要素を成分で書くと、線要素 =
=![\!\left[\begin{array}{c} dx \\ dy \\ dz \end{array}\right]\! $$ \!\left[\begin{array}{c} dx \\ dy \\ dz \end{array}\right]\! $$](./eq/eq-ni-14c1dbc462f83fd3a28dc2e0f4ef3abf.png) 、面要素
、面要素 =
=![\!\left[\begin{array}{c} dydz \\ dzdx \\ dxdy \end{array}\right]\! $$ \!\left[\begin{array}{c} dydz \\ dzdx \\ dxdy \end{array}\right]\! $$](./eq/eq-ni-26b34d17e0dc834680b058a003f9b56b.png) 、体要素
、体要素 =
= 。等式の両辺で
。等式の両辺で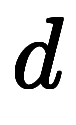 の数が異なるのが問題。微分では
の数が異なるのが問題。微分では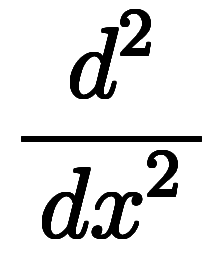 が2階微分を表すように、
が2階微分を表すように、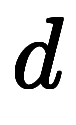 の数は微分の階数。同様に、猫式表記では
の数は微分の階数。同様に、猫式表記では は積分を表し、
は積分を表し、 の数は積分の階数。これら階数が冒頭にあった「次元」の実体で、「次元」を超える置換積分では階数が変化する。このため、微小要素の表記次第で階数が変わっては困る。
の数は積分の階数。これら階数が冒頭にあった「次元」の実体で、「次元」を超える置換積分では階数が変化する。このため、微小要素の表記次第で階数が変わっては困る。
これを回避するため、猫式では微小要素の記号に階数を記入する。 ⇒
⇒ 、
、 ⇒
⇒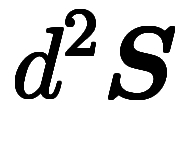 、
、 ⇒
⇒ 。また、それぞれの積分には対応して
。また、それぞれの積分には対応して 、
、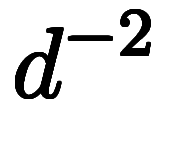 、
、 を使う。ただし、1階の場合は省略して
を使う。ただし、1階の場合は省略して のままも可とする。
のままも可とする。
以上の規則を導入すると、各積分は次のようになる。
| | 分数形 | 演算子形 |
|---|
| 線積分 | \Int[R] \ffd{\b F \sx d^1\b r}{d^1} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ffd {\b F \sx d^1\b r}{d^1}...
l.33 $}
| \Int[R] \ddd{^1\b r}{^1} \sx \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ddd {^1\b r}{^1} \sx \b F } $
l.33 $}
| \Int[R] d^{-1}d^1 \b r \sx \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] d^{-1}d^1 \b r \sx \b F } $
l.33 $}
|
|---|
| 面積分 | \Int[S] \ffd{\b F \sx d^2\b S}{d^2} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {\b F \sx d^2\b S}{d^2}...
l.33 $}
| \Int[S] \ddd{^2\b S}{^2} \sx \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ddd {^2\b S}{^2} \sx \b F } $
l.33 $}
| \Int[R] d^{-2}d^2 \b S \sx \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] d^{-2}d^2 \b S \sx \b F } $
l.33 $}
|
|---|
| 体積分 | \Int[V] \ffd{\b F \, d^3 V}{d^3} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {\b F \, d^3 V}{d^3} } $
l.33 $}
| \Int[V] \ddd{^3 V}{^3} \, \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ddd {^3 V}{^3} \, \b F } $
l.33 $}
| \Int[R] d^{-3}d^3 V \, \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] d^{-3}d^3 V \, \b F } $
l.33 $}
|
|---|
ベクトル置換積分 


猫式では置換積分は次のように書き換わる。
| | 通常表記 | 猫式表記 |
|---|
| ストークスの定理 | \Int[R] \b F \sx d\b r /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \b F \sx d\b r } $
l.33 $}
= \Int[S] \nabla \vx \b F \sx d\b S /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \nabla \vx \b F \sx d\b S } $
l.33 $}
| \Int[R] \ddd{^1\b r \sx}{^1} \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ddd {^1\b r \sx }{^1} \b F } $
l.33 $}
= \Int[S] \ddd{^2\b S \sx}{^2} \ddd{\,\vx}{\b r} \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ddd {^2\b S \sx }{^2} \ddd ...
l.33 $}
|
|---|
| ガウスの定理 | \Int[S] \b F \sx d\b S /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \b F \sx d\b S } $
l.33 $}
= \Int[V] \nabla \sx \b F \, d V /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \nabla \sx \b F \, d V } $
l.33 $}
| \Int[S] \ddd{^2\b S \sx}{^2} \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ddd {^2\b S \sx }{^2} \b F } $
l.33 $}
= \Int[V] \ddd{^3 V }{^3} \ddd{\,\sx}{\b r} \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ddd {^3 V }{^3} \ddd {\,\sx...
l.33 $}
|
|---|
作用対象の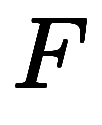 を抜けば、演算子の等価変換となる。
を抜けば、演算子の等価変換となる。
ストークスの定理: \Int[R] \ddd{^1\b r \sx}{^1} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ddd {^1\b r \sx }{^1} } $
l.33 $}
= \Int[S] \ddd{^2\b S \sx}{^2} \ddd{\,\vx}{\b r} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ddd {^2\b S \sx }{^2} \ddd ...
l.33 $}
ガウスの定理: \Int[S] \ddd{^2\b S \sx}{^2} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ddd {^2\b S \sx }{^2} } $
l.33 $}
= \Int[V] \ddd{^3 V }{^3} \ddd{\,\sx}{\b r} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ddd {^3 V }{^3} \ddd {\,\sx...
l.33 $}
演算子としては成立すべき式ではあるが、残念ながらベクトル演算のみでは導けない。したがって、今回の真面目な話はココまで。
イカサマ置換積分 


ここからはふざけててみます。外積代数を習うまでに苦労して開発したイカサマ計算なり。
ガウスの定理の無理な計算 


先に偶然に成立してしまうガウスの定理から。
ガウスの定理の右辺= \Int[V] \ddd{^3V}{^3} \ddd{\,\sx}{\b r} \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ddd {^3V}{^3} \ddd {\,\sx }...
l.33 $}
|
= \Int[V] \ffd{1}{d^3} \, d^3V \left( \ffd{1}{d\b r} \sx d\b F \right) /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^3} \, d^3V \left ...
l.33 $}
= \Int[V] \ffd{1}{d^3} \left(d^3V \ffd{1}{d\b r}\right) \sx d\b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^3} \left (d^3V \f...
l.33 $}
| |
= \Int[V] \ffd{1}{d^3} \!\left(\! \,dxdydz \begin{bmatrix} \ffd1{dx} \\ \ffd1{dy} \\ \ffd1{dz} \end{bmatrix} \!\right)\! \sx d\b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^3} \!\left (\! \,...
l.33 $}
| |
= \Int[V] \ffd{1}{d^3} \!\begin{bmatrix} \ffd{dxdydz}{dx} \\ \ffd{dxdydz}{dy} \\ \ffd{dxdydz}{dz} \end{bmatrix}\! \sx d\b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^3} \!\begin {bmat...
l.33 $}
= \Int[V] \ffd{1}{d^3} \!\begin{bmatrix} dydz \\ dxdz \\ dxdy \end{bmatrix}\! \sx d\b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^3} \!\begin {bmat...
l.33 $}
| |
= \Int[V] \ffd{1}{d^3} \,d^2\b S \sx d\b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^3} \,d^2\b S \sx ...
l.33 $}
| 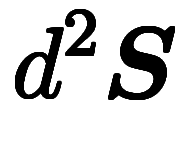 を記号に書き換え を記号に書き換え
|
= \Int[V] \ffd{1}{d^2} \ffd{1}{d}\, d^2\b S \sx d\b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^2} \ffd {1}{d}\, ...
l.33 $}
= \Int[V] \ffd{1}{d^2} d^2\b S \sx \ddd{\b F}{} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^2} d^2\b S \sx \d...
l.33 $}
= \Int[V] \ffd{1}{d^2} d^2\b S \sx F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^2} d^2\b S \sx F } $
l.33 $}
| |
= \Int[V] \ffd{1}{d^2} d^2\b S \sx F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^2} d^2\b S \sx F } $
l.33 $}
= \Int[S] \ffd{1}{d^2} d^2\b S \sx F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d^2\b S \sx F } $
l.33 $}
| |
= \Int[S] \ddd{^2\b S}{^2} \sx F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ddd {^2\b S}{^2} \sx F } $
l.33 $}
=ガウスの定理の左辺 |
ストークスの定理の無理な計算 


続いて、同じ手順をストークスの定理に。
ストークスの定理の右辺= \Int[S] \ddd{^2\b S}{^2} \sx \ddd{\,\vx}{\b r} \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ddd {^2\b S}{^2} \sx \ddd {...
l.33 $}
|
= \Int[S] \ffd{1}{d^2} d^2\b S \sx \left(\ffd{1}{d\b r} \vx d\b F \right) /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d^2\b S \sx \l...
l.33 $}
= \Int[S] \ffd{1}{d^2} d\b F \sx \left(d^2\b S \vx \ffd{1}{d\b r} \right) /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d\b F \sx \lef...
l.33 $}
| |
= \Int[S] \ffd{1}{d^2} d\b F \sx \left( \!\begin{bmatrix} dydz \vphantom{\ddd{}{}} \\ dxdz \vphantom{\ddd{}{}} \\ dxdy \vphantom{\ddd{}{}} \end{bmatrix}\! \vx \!\begin{bmatrix} \ffd1{dx} \\ \ffd1{dy} \\ \ffd1{dz} \end{bmatrix}\! \right) /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d\b F \sx \lef...
l.33 $}
| |
= \Int[S] \ffd{1}{d^2} d\b F \sx \!\begin{bmatrix} dxdz \ffd1{dz} - dxdy \ffd1{dy} \\ dxdy \ffd1{dx} - dydz \ffd1{dz} \\ dydz \ffd1{dy} - dxdz \ffd1{dx} \end{bmatrix}\! /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d\b F \sx \!\b...
l.33 $}
= \Int[S] \ffd{1}{d^2} d\b F \sx \!\begin{bmatrix} dx - dx \\ dy - dy \\ dz - dz \end{bmatrix}\! /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d\b F \sx \!\b...
l.33 $}
| |
= \Int[S] \ffd{1}{d^2} d\b F \sx \b 0 /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d\b F \sx \b 0...
l.33 $}
=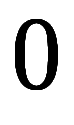 |
はい、外積と除算の結果、ゼロ。
ストークスの定理の無理な計算を通させるイカサマ規則 


上の計算は、実は初っぱなのスカラ三重積からもう成立してない。しかし、逆から計算すれば分かるが、減算の項さえなければ成立する、という非常に惜しい形で破綻している。
このため、
イカサマ規則:微小要素に関わるベクトル演算で、減算項を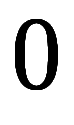 に書き換える。これで、とりあえず計算は通せる。
に書き換える。これで、とりあえず計算は通せる。
そもそも論すらないが、証拠。
⇒ \Int[S] \ffd{1}{d^2} d\b F \sx \!\begin{bmatrix} dxdz \ffd1{dz} - 0 \\ dxdy \ffd1{dx} - 0 \\ dydz \ffd1{dy} - 0 \end{bmatrix}\! /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d\b F \sx \!\b...
l.33 $}
= \Int[S] \ffd{1}{d^2} d\b F \sx \!\begin{bmatrix} dx \\ dy \\ dz \end{bmatrix}\! /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d\b F \sx \!\b...
l.33 $}
| |
= \Int[S] \ffd{1}{d^2} d\b F \sx d\b r /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d\b F \sx d\b ...
l.33 $}
|  を記号に書き換え を記号に書き換え
|
= \Int[S] \ffd{1}{d} \ffd{1}{d}\, d\b F \sx d\b r /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d} \ffd {1}{d}\, d\...
l.33 $}
= \Int[S] \ffd{1}{d} \ffd{d\b F}{d} \sx d\b r /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d} \ffd {d\b F}{d} ...
l.33 $}
= \Int[S] \ffd{1}{d} \b F \sx d\b r /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d} \b F \sx d\b r } $
l.33 $}
| |
= \Int[S] \ffd{1}{d} F \sx d\b r /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d} F \sx d\b r } $
l.33 $}
= \Int[R] \ffd{1}{d} F \sx d\b r /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ffd {1}{d} F \sx d\b r } $
l.33 $}
| |
= \Int[R] \ddd{\b r}{} \sx F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ddd {\b r}{} \sx F } $
l.33 $}
=ストークスの定理の左辺 |
まとめ・つなぎ 


このイカサマ規則は、ストークスの定理を両辺から計算し、違いを吸収するルールとして導入した。目的は、あくまでも3次元ベクトル演算の範囲内で、図を借りずに置換積分を得るだった。上では細かく書いているが、 =
=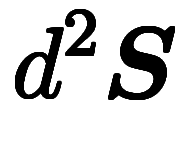 、
、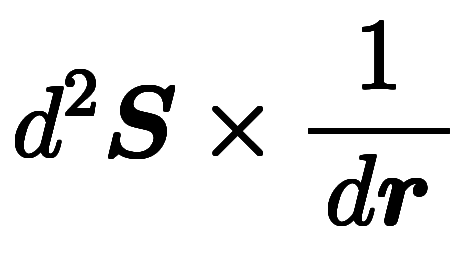 =
= のセンスさえ持っていれば、次のように飛ばせる。
のセンスさえ持っていれば、次のように飛ばせる。
ガウスの定理: \Int[V] \ffd{1}{d^3} d^3V \left( \ffd{1}{d\b r} \sx d \b F \right) /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^3} d^3V \left ( \...
l.33 $}
= \Int[V] \ffd{1}{d^2} \ddd{^3V}{\b r} \sx \ddd{\b F}{} /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[V] \ffd {1}{d^2} \ddd {^3V}{\b ...
l.33 $}
= \Int[S] \ffd{1}{d^2} d^2S \sx \b F /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d^2S \sx \b F } $
l.33 $}
ストークスの定理: \Int[S] \ffd{1}{d^2} d^2\b S \sx \left( \ffd{1}{d\b r} \vx d \b F \right) /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d^2\b S \sx \l...
l.33 $}
= \Int[S] \ffd{1}{d^2} d \b F \sx \left( d^2\b S \vx \ffd{1}{d\b r} \right) /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[S] \ffd {1}{d^2} d \b F \sx \le...
l.33 $}
= \Int[R] \ffd{1}{d } \b F \sx d\b r /home/limg/www/LimgWiki/eq! Undefined control sequence.
$\displaystyle \mathstrut { \Int
[R] \ffd {1}{d } \b F \sx d\b r } $
l.33 $}
さらに、このイカサマも捨てたものではない。実は、ベクトルの次元に関係無く、積分の「次元」を跨がる全ての置換積分に効く。ガウスの定理も減算項が無かったためにイカサマせずに済んだようなもの。微分形式と外積代数から、このイカサマもまた意味を持っていることが分かる。
というわけで、次回は微分形式と外積代数を導入。でも、タイトルは基底成分表記(仮)。
![[PukiWiki] [PukiWiki]](image/NekoPunch.png)
![[PukiWiki] [PukiWiki]](image/NekoPunch.png)



 =
=![\!\left[\begin{array}{c} dx \\ dy \\ dz \end{array}\right]\! $$ \!\left[\begin{array}{c} dx \\ dy \\ dz \end{array}\right]\! $$](./eq/eq-ni-14c1dbc462f83fd3a28dc2e0f4ef3abf.png) 、面要素
、面要素 =
=![\!\left[\begin{array}{c} dydz \\ dzdx \\ dxdy \end{array}\right]\! $$ \!\left[\begin{array}{c} dydz \\ dzdx \\ dxdy \end{array}\right]\! $$](./eq/eq-ni-26b34d17e0dc834680b058a003f9b56b.png) 、体要素
、体要素 =
= 。等式の両辺で
。等式の両辺で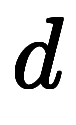 の数が異なるのが問題。微分では
の数が異なるのが問題。微分では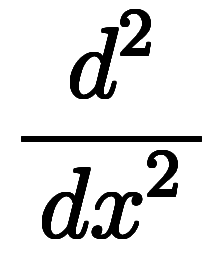 が2階微分を表すように、
が2階微分を表すように、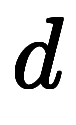 の数は微分の階数。同様に、猫式表記では
の数は微分の階数。同様に、猫式表記では は積分を表し、
は積分を表し、 の数は積分の階数。これら階数が冒頭にあった「次元」の実体で、「次元」を超える置換積分では階数が変化する。このため、微小要素の表記次第で階数が変わっては困る。
の数は積分の階数。これら階数が冒頭にあった「次元」の実体で、「次元」を超える置換積分では階数が変化する。このため、微小要素の表記次第で階数が変わっては困る。 ⇒
⇒ 、
、 ⇒
⇒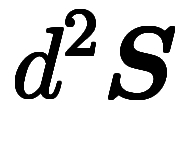 、
、 ⇒
⇒ 。また、それぞれの積分には対応して
。また、それぞれの積分には対応して 、
、 、
、 を使う。ただし、1階の場合は省略して
を使う。ただし、1階の場合は省略して のままも可とする。
のままも可とする。


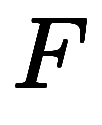 を抜けば、演算子の等価変換となる。
を抜けば、演算子の等価変換となる。











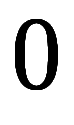 に書き換える。これで、とりあえず計算は通せる。
に書き換える。これで、とりあえず計算は通せる。


 =
=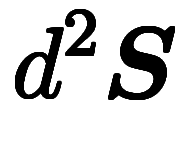 、
、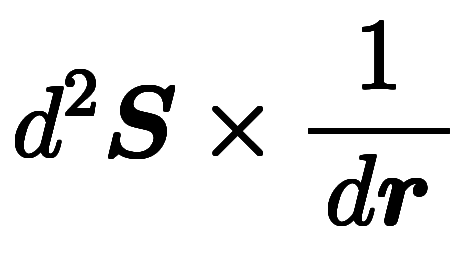 =
= のセンスさえ持っていれば、次のように飛ばせる。
のセンスさえ持っていれば、次のように飛ばせる。